We use cookies to ensure you have the best browsing experience on our website. Please read our cookie policy for more information about how we use cookies.
  1. Prepare
  2. Algorithms
  3. Graph Theory
  4. Journey Scheduling

Journey Scheduling

Fedya is a seasoned traveller and is planning his trip to Treeland. Treeland is a country with an ancient road system which is in the form of a tree structure. cities of Treeland are numbered by positive integers: .

Fedya has not yet decided the starting point (city) of his journey and the cities he will visit. But there are a few things you know about Fedya's trip:

  • Fedya is fond of travelling to great distances. So if he is currently located in city , his destination will be a city which is most distant from city .

  • There might be more than 1 such cities. In that case, Fedya will choose a city that was already visited as less times as possible in this journey.

  • There still might be more than 1 such cities. In that case, Fedya will go to the city with the smallest number.

Fedya has prepared a list of possible journeys. Each one is characterized by two integers - the starting city and the total number of cities to be visited, . For each of them, he is keen to know the total distance travelled by him.

Input Format

The first line of input will contain two space separated integers and - the number of cities and the number of possible journeys.

Then, there will be lines, each of them will contain two space separated integers , denoting the bi-directional road between the cities with numbers and with the unitary length.

Then there will be lines, each of them will have two space separated integers and , denoting a journey.

Constraints



Output Format

For each journey, output the travelled distance on a separate line.

Sample Input

8 7
2 1
3 2
4 2
5 1
6 1
7 1
8 7
4 6
3 4
6 3
7 6
4 6
7 1
2 6

Sample Output

24
16
11
23
24
3
23

Explanation

The tree in question is given in the picture below.

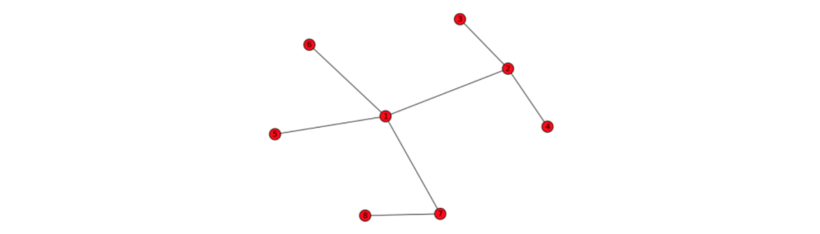
image

  • 4 6 indicates that Fedya starts at 4. Now we see that the most distant city from 4 is 8. Fedya now travels to city 8. From 8, the most distance cities are [4, 3]. As 4 is already visited, he chooses to visit city 3. From city 3, he revisits city 8 and so on. The cities in the order of visit is 4 - > 8 -> 3 -> 8 -> 4 -> 8 -> 3 which sums to 24. Hence, the answer.
  • 6 3 indicates that Fedya starts at city 6. From 6, the most distant cities are [3,4,8]. In this leg of the journey, no city is visited and hence Fedya chooses to visit the city with the smallest number 3. From 3, he visits 8 and then he ends his trip at city 4 which sums to 3 + 4 + 4 = 11. Hence, the answer.